How To Learn Calculus In A Week

I have a love/detest relationship with calculus: it demonstrates the beauty of math and the agony of math education.

Calculus relates topics in an elegant, brain-bending manner. My closest analogy is Darwin's Theory of Development: once understood, you beginning seeing Nature in terms of survival. Yous sympathise why drugs lead to resistant germs (survival of the fittest). You know why saccharide and fat gustation sweet (encourage consumption of high-calorie foods in times of scarcity). It all fits together.

Calculus is similarly enlightening. Don't these formulas seem related in some way?

circle sphere formula

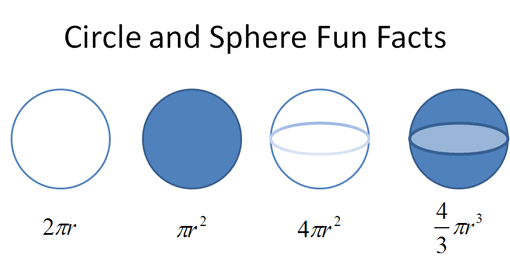
They are. But about of us learn these formulas independently. Calculus lets united states kickoff with $\text{circumference} = 2 \pi r$ and effigy out the others — the Greeks would accept appreciated this.

Unfortunately, calculus tin can recap what'southward wrong with math educational activity. Most lessons feature contrived examples, arcane proofs, and memorization that torso slam our intuition & enthusiasm.

Information technology really shouldn't be this way.

Math, art, and ideas

I've learned something from schoolhouse: Math isn't the difficult part of math; motivation is. Specifically, staying encouraged despite

Teachers focused more than on publishing/perishing than education
Cocky-fulfilling prophecies that math is difficult, tedious, unpopular or "non your subject"
Textbooks and curriculums more concerned with profits and test results than insight

'A Mathematician'south Lament' [pdf] is an excellent essay on this issue that resonated with many people:

"…if I had to blueprint a machinery for the express purpose of destroying a kid'due south natural curiosity and love of pattern-making, I couldn't peradventure practice as skillful a chore equally is currently being done — I simply wouldn't have the imagination to come up up with the kind of senseless, soul-crushing ideas that constitute contemporary mathematics education."

Imagine pedagogy art like this: Kids, no fingerpainting in kindergarten. Instead, let's report pigment chemical science, the physics of light, and the anatomy of the eye. After 12 years of this, if the kids (now teenagers) don't hate fine art already, they may begin to kickoff coloring on their own. After all, they have the "rigorous, testable" fundamentals to start appreciating art. Right?

Poetry is like. Imagine studying this quote (formula):

"This above all else: to thine own cocky exist true, and it must follow, as nighttime follows solar day, thou canst not and then be simulated to whatsoever human." —William Shakespeare, Hamlet

It's an elegant manner of proverb "exist yourself" (and if that means writing irreverently about math, so be it). But if this were math class, nosotros'd be counting the syllables, analyzing the iambic pentameter, and mapping out the field of study, verb and object.

Math and poetry are fingers pointing at the moon. Don't confuse the finger for the moon. Formulas are a means to an end, a fashion to express a mathematical truth.

We've forgotten that math is about ideas, not robotically manipulating the formulas that express them.

Ok bub, what'southward your great idea?

Feisty, are nosotros? Well, hither'southward what I won't practice: recreate the existing textbooks. If y'all need answers right away for that large test, in that location'due south plenty of websites, course videos and xx-infinitesimal sprints to aid yous out.

Instead, allow'southward share the core insights of calculus. Equations aren't enough — I want the "aha!" moments that make everything click.

Formal mathematical language is one simply ane mode to communicate. Diagrams, animations, and simply plain talkin' can frequently provide more than insight than a page total of proofs.

But calculus is hard!

I remember anyone can appreciate the core ideas of calculus. Nosotros don't need to be writers to enjoy Shakespeare.

It's inside your accomplish if you know algebra and have a full general interest in math. Not long ago, reading and writing were the work of trained scribes. Withal today that can be handled past a x-year old. Why?

Considering nosotros expect it. Expectations play a huge part in what'due south possible. So expect that calculus is only another subject. Some people get into the nitty-gritty (the writers/mathematicians). But the rest of us tin can still adore what's happening, and expand our brain forth the way.

It's about how far you want to go. I'd love for everyone to understand the core concepts of calculus and say "whoa".

So what'southward calculus about?

Some define calculus as "the co-operative of mathematics that deals with limits and the differentiation and integration of functions of one or more variables". Information technology's correct, but non helpful for beginners.

Here's my take: Calculus does to algebra what algebra did to arithmetics.

Arithmetic is virtually manipulating numbers (addition, multiplication, etc.).
Algebra finds patterns between numbers: $a^2 + b^two = c^2$ is a famous relationship, describing the sides of a right triangle. Algebra finds unabridged sets of numbers — if y'all know a and b, you lot tin can observe c.
Calculus finds patterns between equations: you lot can see how one equation ($\text{circumference} = 2 \pi r$) relates to a similar one ($\text{area} = \pi r^2$).

Using calculus, we can ask all sorts of questions:

How does an equation grow and shrink? Accumulate over time?
When does information technology accomplish its highest/everyman point?
How practice nosotros use variables that are constantly changing? (Rut, move, populations, …).
And much, much more than!

Algebra & calculus are a trouble-solving duo: calculus finds new equations, and algebra solves them. Similar evolution, calculus expands your understanding of how Nature works.

An Example, Delight

Let's walk the walk. Suppose nosotros know the equation for circumference ($2 \pi r$) and want to find surface area. What to do?

Realize that a filled-in disc is like a set of Russian dolls.

Disc and Rings

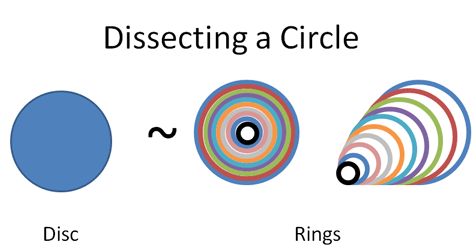
Hither are two ways to draw a disc:

Make a circle and fill it in
Draw a bunch of rings with a thick marker

The amount of "infinite" (area) should be the same in each case, right? And how much space does a ring use?

Well, the very largest ring has radius "r" and a circumference $2 \pi r$. As the rings go smaller their circumference shrinks, only it keeps the pattern of $2 \pi \cdot \text{current radius}$. The final ring is more like a pinpoint, with no circumference at all.

Disc and Ring Area

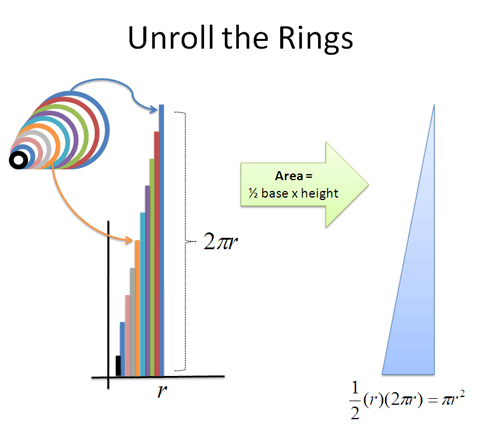
Now here's where things get funky. Let'southward unroll those rings and line them up. What happens?

Nosotros get a bunch of lines, making a jagged triangle. But if we take thinner rings, that triangle becomes less jagged (more on this in futurity articles).
One side has the smallest band (0) and the other side has the largest band ($2 \pi r$)
Nosotros have rings going from radius 0 to upward to "r". For each possible radius (0 to r), we just place the unrolled ring at that location.
The total surface area of the "band triangle" = $\frac{one}{2} \text{ base of operations} \cdot \text{height} = \frac{ane}{2} (r) (2 \pi r) = \pi r^ii$, which is the formula for area!

Yowza! The combined surface area of the rings = the expanse of the triangle = area of circle!

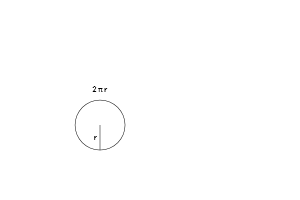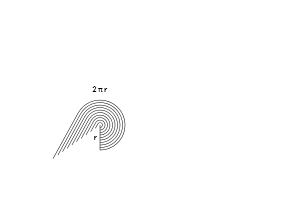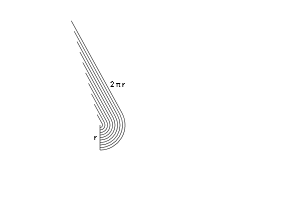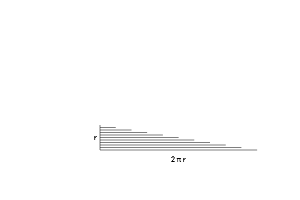
Triangle from circle

(Prototype from Wikipedia)

This was a quick example, just did you catch the key thought? We took a disc, carve up it up, and put the segments together in a different way. Calculus showed u.s.a. that a disc and band are intimately related: a disc is really merely a bunch of rings.

This is a recurring theme in calculus: Big things are made from little things. And sometimes the piddling things are easier to work with.

A annotation on examples

Many calculus examples are based on physics. That's great, just information technology can exist hard to relate: honestly, how often do you know the equation for velocity for an object? Less than one time a week, if that.

I prefer starting with physical, visual examples because it's how our minds work. That ring/circle matter we made? You could build information technology out of several pipe cleaners, separate them, and straighten them into a crude triangle to see if the math really works. That's simply not happening with your velocity equation.

A note on rigor (for the math geeks)

I can feel the math pedants firing up their keyboards. Just a few words on "rigor".

Did you know nosotros don't learn calculus the way Newton and Leibniz discovered it? They used intuitive ideas of "fluxions" and "infinitesimals" which were replaced with limits because "Sure, information technology works in practice. But does information technology piece of work in theory?".

We've created complex mechanical constructs to "rigorously" show calculus, but have lost our intuition in the procedure.

We're looking at the sweetness of sugar from the level of brain-chemistry, instead of recognizing information technology as Nature's way of saying "This has lots of energy. Swallow it."

I don't want to (and tin can't) teach an analysis course or railroad train researchers. Would information technology be so bad if everyone understood calculus to the "non-rigorous" level that Newton did? That it inverse how they saw the world, every bit information technology did for him?

A premature focus on rigor dissuades students and makes math hard to acquire. Case in point: due east is technically defined by a limit, only the intuition of growth is how information technology was discovered. The natural log tin can exist seen as an integral, or the time needed to grow. Which explanations assistance beginners more than?

Let'due south fingerpaint a bit, and go into the chemistry along the way. Happy math.

(PS: A kind reader has created an animated powerpoint slideshow that helps present this idea more visually (all-time viewed in PowerPoint, due to the animations). Thanks!)

Notation: I've made an entire intuition-beginning calculus serial in the style of this article:

https://betterexplained.com/calculus/lesson-1